Page 9 - Shimadzu DUH-211/

Data Processing ISO 14577-1 (Annex A) Compliant Evaluation
(Instrumented Indentation Test for Hardness)

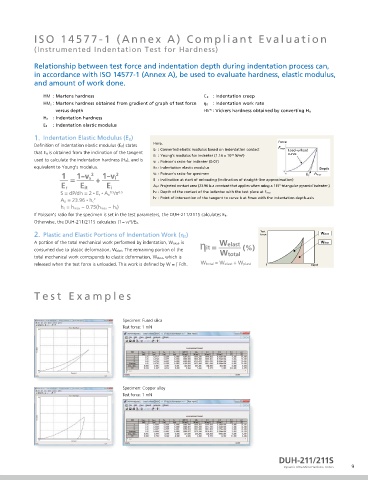
Simply set the required items to obtain the desired information. Relationship between test force and indentation depth during indentation process can,
in accordance with ISO 14577-1 (Annex A), be used to evaluate hardness, elastic modulus,
and amount of work done.
Data Processing Items
· Results display HM : Martens hardness Cit : Indentation creep
· Data output for test force and depth HMs : Martens hardness obtained from gradient of graph of test force ηit : Indentation work rate
· Graph output for test force and depth versus depth HV* : Vickers hardness obtained by converting Hit
: Indentation hardness
· Graph output for hardness and depth Hit
Eit : Indentation elastic modulus
· Graph output for hardness between 2 points and depth
· Graph output for depth and time 1. Indentation Elastic Modulus (E it)
· Graph output for hardness and test force Definition of indentation elastic modulus (Eit) states Here, Force
Er : Converted elastic modulus based on indentation contact F max Load–unload
· Graph output for depth squared and test force that Eit is obtained from the inclination of the tangent curve
Ei : Young's modulus for indenter (1.14 × 10 12 N/m 2 )
· Hardness calculation based on preliminary test force used to calculate the indentation hardness (Hit), and is νi : Poisson's ratio for indenter (0.07)
· Graph output for hardness and parameters equivalent to Young's modulus. Eit : Indentation elastic modulus Depth
1
2
2
νs : Poisson's ratio for specimen
· Calculation of converted hardness values - - - S : Inclination at start of unloading (inclination of straight-line approximation) h r h max
+
1−ν i
1−ν s
=
· Repeated changes of surface detection points E r E it E i Ap : Projected contact area (23.96 is a constant that applies when using a 115° triangular pyramid indenter.)
0.5
· Calculation of elastic modulus S = dP/dh = 2 · E r · A p /π 0.5 hc : Depth of the contact of the indenter with the test piece at Fmax
2 hr : Point of intersection of the tangent to curve b at Fmax with the indentation depth-axis
· ASCII file output A p = 23.96 · h c
h c = h max − 0.75(h max − h r)
If Poisson's ratio for the specimen is set in the test parameters, the DUH-211/211S calculates Eit.
2
Otherwise, the DUH-211/211S calculates (1− νS )/Eit.
2. Plastic and Elastic Portions of Indentation Work (η it) Test Wplast
force
A portion of the total mechanical work performed by indentation, Wtotal, is Welast Welast
ηit = - (%)
consumed due to plastic deformation, Wplast. The remaining portion of the
Example of test results display (load–unload test) Wtotal
total mechanical work corresponds to elastic deformation, Welast, which is
released when the test force is unloaded. This work is defined by W = ∫ Fdh. W total = W elast + W plast 0 Depth
Test Examples

Specimen: Fused silica
Test force: 1 mN

Graph of depth squared against test force

Specimen: Copper alloy
Test force: 1 mN

Calculation of elastic modulus

DUH-211/211S
8 Dynamic Ultra Micro Hardness Testers 9